Muskan Shafi Education Content Expert
Education Content Expert
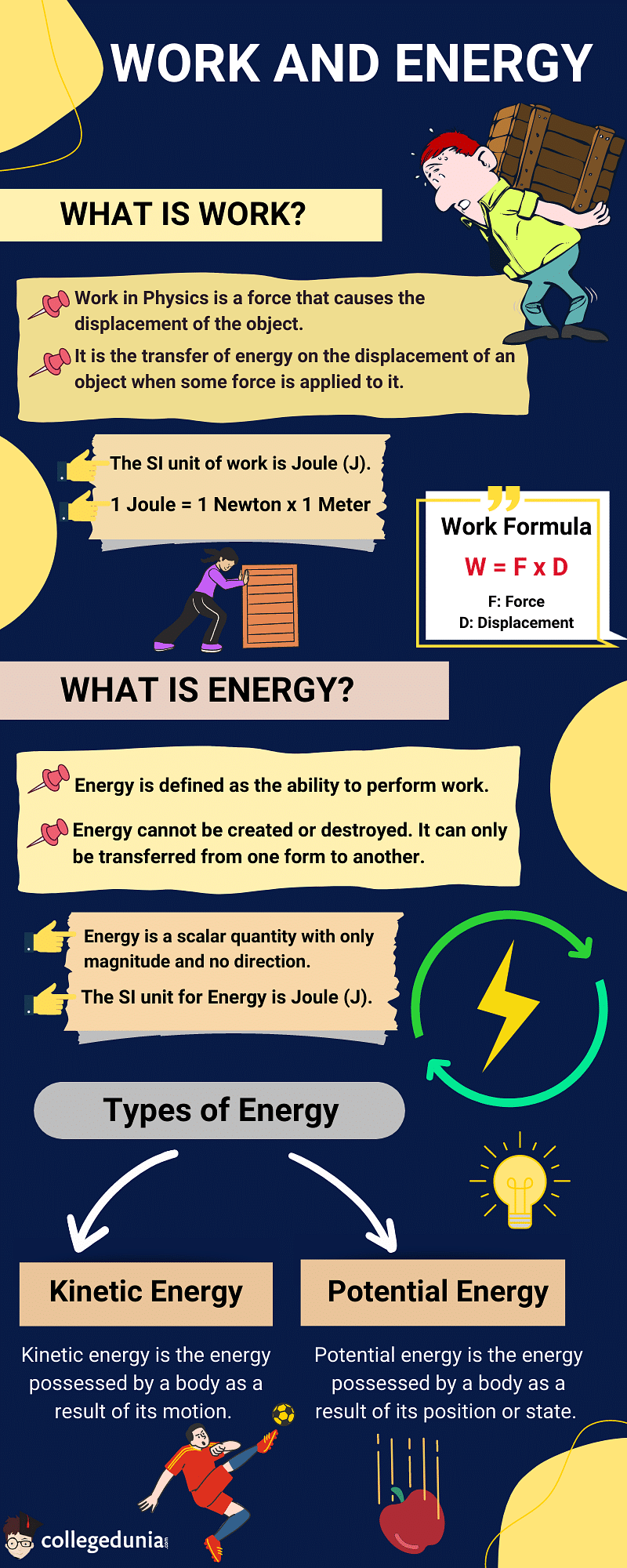
Work and Energy are the two fundamental concepts of Physics that are closely related to each other. Work is the transfer of energy that occurs when an object is moved over a distance by an external force. Energy is defined as the capacity to do work.
- Work refers to the displacement of an object when a force is applied to it.
- It is the product of the force applied and displacement.
- Work is calculated as W = F.d, where F is the force and d is the displacement.
- Energy is the ability to perform to do work or perform tasks.
- It can be changed from one form to another, but it can never be created or destroyed.
Read More: NCERT Solutions for Class 11 Physics Work, Energy, and Power
Key Terms: Work, Energy, Work Done, Work-Energy Theorem, Displacement, Force, Kinetic Energy, Potential Energy, Work Formula
What is Work?
[Click Here for Sample Questions]
Work in Physics is a force that causes the displacement of the object. It is a measure of the transfer of energy on the displacement of an object when some force is applied to it.
- It is the product of the force applied and the magnitude of the displacement.
- It is denoted by the symbol ‘W’.
- The SI unit of work is Joule (J).
- Work is a scalar quantity with only magnitude and no direction.

Work done can be positive, negative, or zero.
Positive Work
Work is said to be positive when both displacement and force are in the same direction. Force and displacement act in the same direction when an object moves on a horizontal surface. As a result, the work done is positive.

Positive Work
Negative Work
Work done is said to be negative when both displacement and force are in the opposite direction. The force of gravity acts in a downward direction when an object is thrown upwards, whereas displacement acts in an upward direction, hence the work done is negative.

Zero Work
If there is no displacement in the object, there is no work done. Also, if the force acting on the body is zero or force and displacement are mutually perpendicular, then the work done is zero.

Zero Work
Read More:
Unit of Work
[Click Here for Previous Years’ Questions]
Joule (J) is the SI unit of work. One joule is the energy required to accelerate 1 kg of a body by a force of 1 N over a distance of 1m.
| 1 Joule = 1 Newton x 1 Meter |
The CGS unit of work is erg (dyn cm). It is also measured in units like erg, the foot-pound, foot-poundal, kilowatt-hour, liter-atmosphere, and horsepower-hour.
Conversion of Units of Work
The conversion of the various units of work is as follows:
| Unit | Conversion |
|---|---|
| British Thermal Unit (BTU) | 1055.06 Joule |
| 1 erg | 10-7 Joule |
| 1 horsepower-hour | 2684519.5377 Joule |
| 1 foot-pound | 1.36 Joule |
| 1 Kilo-watt Hour | 3.6 million Joule |
| 1 Newton-meter | 1 Joule |
Read More: Difference between Work and Energy
Work and Energy
Work Formula
[Click Here for Sample Questions]
Work is the product of the force and displacement of the object. Work Formula is given as
| W = F.d |
Work Formula in the case when the force is been exerted at an angle θ to the displacement is as follows:
| W = F.d.cos θ |
Where
- W is the Work Done by the object.
- F is the Force Applied to the object.
- d is the Displacement in the direction of the force.
- θ is the angle between force and displacement.

Read More: Work, Energy and Power Important Questions
What is Energy?
[Click Here for Previous Years’ Questions]
Energy is defined as the ability to perform work. Energy cannot be created or destroyed. It can only be transferred from one form to another.
- Energy is a scalar quantity with only magnitude and no direction.
- The SI unit for energy is Joule (J).
- The suns and the stars are the most abundant sources of energy.
- It is affected by position, mass, speed, shape, and various other factors.
Read More: Geothermal Energy
Types of Energy
[Click Here for Sample Questions]
Energy can be categorized into two major types namely
- Kinetic Energy
- Potential Energy
Kinetic Energy
Kinetic energy is the energy possessed by a body as a result of its motion. It is the amount of work necessary to accelerate a body of a specific mass from rest to a given velocity.
Kinetic Energy Formula is given as
| K.E. = ½ mv2 |
Where
- KE: Kinetic Energy.
- m: Mass of the Object.
- v: Velocity of the Object.
Potential Energy
Potential energy is the energy possessed by a body as a result of its position or state. Potential energy is further divided into two types:
- Gravitational Potential Energy
- Elastic Potential Energy
Gravitational Potential Energy
The energy possessed by a body as a result of its position above the earth's surface is known as gravitational potential energy. The formula for calculating Gravitational Potential Energy is
| P.E. = mgh |
Where
- m: Mass of a body.
- g: Acceleration due to gravity on the surface of the earth.
- h: Height through which the body is raised.
Elastic Potential Energy
Work must be done against the restoring elastic force when an elastic body is pushed from its equilibrium position. The work that is done is stored in the body as elastic potential energy. The elastic potential energy of an elastic spring is given if it is stretched (or compressed) by a distance Y from its equilibrium position.
| U = ½ kx2 |
Where
- U: Elastic Potential Energy.
- k: Spring Force Constant.
- x: Length of String Stretch.
Read More: Difference between Kinetic and Potential Energy
Work Energy Theorem
[Click Here for Previous Years’ Questions]
Work-Energy Theorem is derived from the Law of Conservation of Energy.
According to the work-energy theorem,
| “The change in kinetic energy of an object is equal to the net work done on the object.” |
It can be represented as:
| W = Kf – Ki |
Where
- Kf: Final Kinetic Energy
- Ki: Initial Kinetic Energy
- W: Net Work Done
Read More: Work, Energy and Power MCQs
Work and Energy Equations
[Click Here for Sample Questions]
Work is the resultant product of the force applied (F) on the object and the displacement (d). It is calculated as
| W = F.d |
Work done against gravity is calculated as
| W = m x g x h |
Where
- W: Work Done
- m: Mass of Object
- g: Acceleration due to Gravity
- h: Height through which the object is been lifted.
Potential Energy and Kinetic Energy are the two types of energy.
| K.E. = ½ mv2 |
| P.E. = mgh |
Check More:
Difference Between Work and Energy
[Click Here for Previous Years’ Questions]
Work and Energy are two closely-related concepts in Physics. The key difference between work and energy is as follows:
| Work | Energy |
|---|---|
| Work is defined as the process of transferring energy into an object in order to cause displacement. | The ability to work is defined as energy. |
| The work that is completed is always the same. | There are several types of energy, including kinetic and potential energy. |
| W = F.d is the mathematical expression of work, where F is the applied force and d represents the object's displacement. | KE = ½ mv2 is the kinetic energy formula, while PE = mgh is the potential energy formula, where m is the mass, v is the velocity, g is the acceleration due to gravity, and h is the height. |
Things to Remember
- Work is said to be done when displacement occurs due to the application of force on an object.
- Work done is defined as the product of the force and displacement.
- Work Formula is given as W = F x d.
- Work done can be positive, negative, or even zero.
- Joule is the SI unit of work (J).
- Energy is the ability to accomplish tasks.
- According to the Law of Conservation of Energy, energy cannot be created or destroyed.
- There are two main types of energy namely kinetic energy and potential energy.
- According to the work-energy theorem, the net work done on an object is equal to the change in the object's kinetic energy. theorem.
Previous Years’ Questions
- When a long spring is stretched by 2cm, its potential….[NEET 2003]
- The kinetic energy of a body of mass 4 kg….[KCET 2015]
- The kinetic energy of a body becomes four times….[KCET 2000]
- A gun fires a small bullet with kinetic….[KCET 2013]
- A motor pump lifts 6 tonnes of water from a well of depth 25m….[KCET 2017]
- The work done by a force acting on a body is as shown in….[KCET 2009]
- During an inelastic collision between two bodies...[KCET 2019]
- What is the work done to drag the 60 kg weight...
- Calculate the work done by the applied force... [DUET 2011]
- Calculate the work done when the spring is stretched from 0.1 to 0.2 m...
- What is the work done in taking charge of Q once along the loop... [NEET 2005]
- Calculate the work done by the force in displacing the particle... [KEAM]
- A batsman hits back a ball straight in the direction of the… (NEET 1989)
- A vertical spring with force constant k is fixed on a table. A ball… (NEET 2007)
- A spring stores 1J of energy for compression of… (KEAM)
Sample Questions
Ques. What will be the work done if 20 N of force is applied on an object to cause a displacement of 3 m? (3 Marks)
Ans. It is given that
- Force Applied (F) = 20 N
- Displacement (D) = 3 m
Using the Work Formula,
W = F.d
W = 20 × 3
W = 60 Joules
Thus, the work done is 60 joules.
Ques. What will be the kinetic energy of a 500kg car moving at a speed of 36km/h? (3 Marks)
Ans. It is given that,
- Mass m = 500 kg
- Velocity v = 36km/hr = 10m/s
Using the Kinetic Energy Formula,
K = ½ mv2
K = ½ x 500 x 10 x 10 = 25000 J
Thus, the kinetic energy of the car is 25000 J.
Ques. If a ball of mass 5 kg is placed on a higher ground of 3 meters, find the potential energy stored in that body. (3 Marks)
Ans. Given that
- m = 5 kg
- h = 3 m
- g = 9.81 m/s-2
Using the Potential Energy Formula,
Potential Energy = mgh
PE = 5 x 3 x 9.81
PE = 147.15 J
Thus, the potential energy of the ball is 147.15 J.
Ques. A cyclist comes to a skidding stop at 10 m. During this process, the force on the cycle due to the road is 200 N and is directly opposed to the motion. (a) How much work does the road do on the cycle? (b) How much work does the cycle do on the road? (3 Marks)
Ans. Work done on the cycle by the road is the work done by the stopping (frictional) force on the cycle due to the road.
(a) The stopping force and the displacement make an angle of 180 degrees (π rad) with each other. Thus, work done by the road,
W = Fd cosθ = 200 × 10 × cos π = – 2000 J
It is this negative work that brings the cycle to a halt in accordance with the work-energy theorem.
(b) From Newton’s Third Law of motion, an equal and opposite force acts on the road due to the cycle. Its magnitude is 200 N. However, the road undergoes no displacement. Thus, work done by cycle on the road is zero.
Ques. What is the unit of Work and Energy? (2 Marks)
Ans. Joule (J) is the SI unit of both work and energy. One joule is defined as the energy needed to accelerate 1 kg of a body by a force of 1 N over a distance of 1m.
1 Joule = 1 Newton x 1 Meter
Ques. State, whether the following work done, are positive or negative:
(a) Work done by a man in lifting a bucket out of a well by means of a rope tied to the bucket.
(b) Work done by the gravitational force in the above case.
(c) Work done by friction on a body sliding down an inclined plane.
(d) Work done by an applied force on a body moving on a rough horizontal plane with uniform velocity.
(e) Work done by the resistive force of air on a vibrating pendulum in bringing it to rest. (5 Marks)
Ans. It is known that, Work done, W = Fd cos θ.
(a) Work done ‘positive’, because force is acting in the direction of displacement i.e., θ = 0°.
(b) Work done is negative, because force is acting against the displacement i.e., θ = 180°.
(c) Work done is negative because the force of friction is acting against the displacement i.e., θ= 180°.
(d) Work done is positive, because the body moves in the direction of applied force i.e., θ= 0°.
(e) Work done is negative, because the resistive force of air opposes the motion i.e., θ = 180°.
Ques. An electric heater is rated 1500 W. How much energy does it use in 10 hours? (3 Marks)
Ans. Energy consumed by an electric heater can be obtained with the help of the expression,
P = W/t
Where
- Power rating of the heater, P = 1500 W = 1.5 kW
- Time for which the heater has operated, t = 10 h
Work done = Energy consumed by the heater
Therefore, energy consumed = Power × Time = 1.5 × 10 = 15 kWh
Hence, the energy consumed by the heater in 10 h is 15 kWh or 15 units.
Ques. Find the energy in kWh consumed in 10 hours by four devices of power 500 W each. (3 Marks)
Ans. Given that,
- Power rating of each device, P = 500 W = 0.50 kW
- Time for which each device runs, t = 10 h
- Work done = Energy consumed by each device (E)
Using the Power Formula,
Power = Energy Consumed / Time
Energy consumed by each device= Power × Time
E = P x t
E = 0.50 × 10 = 5 kW/h
Hence, the energy consumed by four devices of power 500 W each in 10 h will be
4 × 5 kWh = 20 kWh = 20 units
Ques. Determine the work done if a force of 30 N acts on an object and causes a displacement of 8 m in the direction of the force. (3 Marks)
Ans. According to the question,
- Force (F) = 30 N
- Displacement (d) = 8 m
Using the Work Formula,
W = Fd
W = 30 x 8
W = 240 J
Thus, the work done is calculated as 240 J.
Ques. When is work done considered to be zero? (1 Mark)
Ans. Work done is considered to be zero if the direction of the force and the displacement are perpendicular to each other. For instance, if we push forcefully against a wall, the force we are exerting on the wall is ineffective since the wall’s displacement is zero.
Check-Out:





Comments