
Content Curator
Universal Law of gravitation came into existence in the year 1687 by Sir Issac Newton. The law provided reasoning to the motion of the planets and their satellites. Gravity, also known as Gravitation, is one of the four universal forces of attraction that helps to keep things together. It is this gravitational force that holds us onto the Earth and does not let us fly up into space. Universal law of gravitation gives the relation between the attraction of two particles considering their mass and distance between them.
Any two objects that posses non-zero mass have a force of gravity that attracts them towards each other. The gravitational force acts on any object irrespective of its shape, size, or composition. Attraction of objects in outer space or falling of an object when it is thrown upwards are examples of gravitation in our everyday life.
Also Read: Value of g on Moon
| Table of Content |
Key Terms: Universal law of Gravitation, Gravity, Gravitation, Value of Gravitational Constant, Gravitational Force, Newton’s Law of Gravitation
What is Gravitation?
[Click Here for Sample Questions]
- Gravitation or gravity is an all-pervasive universal gravitational force that attracts a body towards the centre of the Earth or any other physical body having a mass.
- Along with Electromagnetism and strong and weak nuclear forces, Gravitation constitutes the four fundamental forces of nature.
- Although gravitational force is the weakest known force of the four but is absolutely crucial as it controls all the trajectories of the bodies in the universe and the cosmos.
Understanding Gravity & Gravitation
Read More: What if the Earth Stopped Spinning?
Universal Law of Gravitation
[Click Here for Sample Questions]
The universal law of gravitation states that
Every particle of matter in the universe attracts any other particle with a gravitational force directly proportional to the product of the masses and inversely proportional to the square of the distance between them.
This can be mathematically represented as:
\(F\propto \frac{m_1m_2}{r^2}\)
Removing the proportionality and replacing with a constant we can rewrite as
\(F= G \frac{m_1m_2}{r^2}\)
Where,
F- Force acting on the object
m1 and m2 - mass of the objects
r- distance between the objects
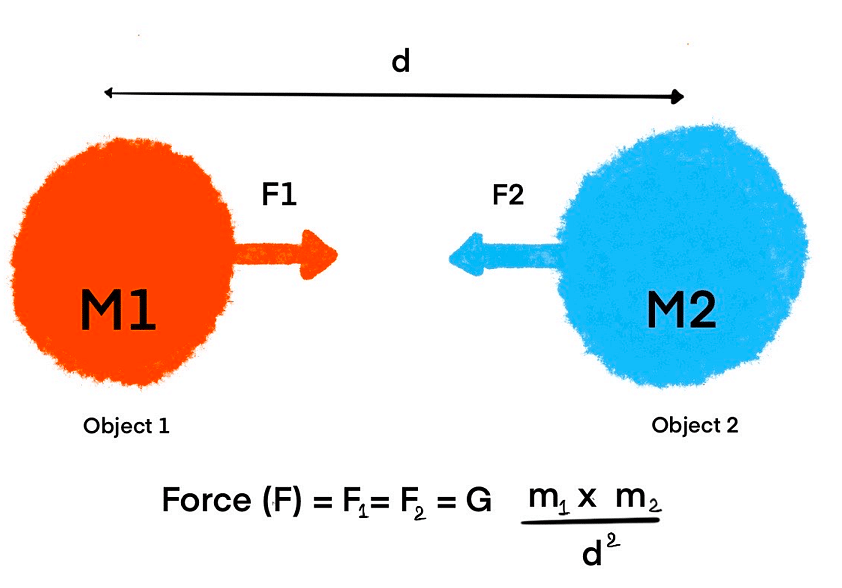
Universal Law of Gravitation
Universal Equation of Gravitation
Mathematically, it can be written as,
F∝m1m2…………………………….(i)
and F∝1/r2…………………………………(ii)
Combining the two equations we get,
F∝m1m2/ r2
\(F= G \frac{m_1m_2}{r^2}\)
Read More: Value of g
Importance of Gravitational Force
[Click Here for Sample Questions]
- Gravitational force is a conservative force.
- It acts along the centre of the line joining two objects together and are called central forces.
- It is independent of the medium between the two objects.
- It is independent of the nature and size of the body.
- Gravitational force follows the Newton’s third law of motion and forms an action-reaction pair.
- it is not dependent on the presence or absence of other bodies in the surrounding environement.
Gravitational Constant
[Click Here for Sample Questions]
- Universal gravitational constant, also known as Newton’s gravitational Constant or Cavendish gravitational Constant.
- It is an empirical constant, proven through a series of experiments, involved in the mathematical computations of gravitational effects in Newton’s Laws of gravitation and Einstein’s general theory of relativity.
- The first implicit measurement of the gravitational constant was measured by Henry Cavendish in 1798.
- Gravitational force of attraction between any two-unit masses separated by unit distance.
- In Newton’s law, it is the proportional constant that is used to connect the gravitational forces between two bodies having mass where it is the product of their masses and inverse square of their distance.
- Einstein’s field equation quantifies the relationship between the geometry of space-time and the stress-energy tensor.
- The gravitational constant is denoted by ‘G’ and its value is 6.674×10−11 m3⋅kg−1⋅s−2 .
Check Out:
Weight and Gravitational Force
[Click Here for Sample Questions]
From Newton’s law of Gravitation, we notice that mass is a very important entity. Although we often consider the mass and the weight synonymously, in reality, they are significantly different.
- An object’s weight can be derived by multiplying that object’s mass with the acceleration due to gravity (g) at Earth surface.
- However, the value of ‘g’ changes from place to place.
- The value of ‘g’ of the earth has been measured at 9.8 m/s2.
- It can be mathematically written as
W = mg
where, W= weight of the object, m= mass of the object and g= acceleration of the object due to gravity.
- The gravitational force is simply the attraction force between two masses.

Difference between Mass & Weight
According to the Universal law of gravity,
F= Gm1m2/ r2………… (iii)
Newton’s Second law of motion states,
g= F/m …………(iv)
Substituting equation (iii) in (iv) we get-
g= Gm1m1/r2m
Thus, we get the formula of 'g’
g = Gm1/r2
Here,
g = acceleration due to the gravity of the heavier body in m/s2
G = universal gravitational constant in Nm2/kg2
r = the radius of the heavier body in km
m1 = the mass of the heavier body in Kg
Note: G and g are independent of each other.
Read Also: Free Fall Formula
Universality of Gravity
[Click Here for Sample Questions]
- Gravitation is considered to be a force that is acting only between the massive celestial bodies.
- In reality, gravitation is the universal force of attraction that helps to keep things together.
- It exists between all the bodies having mass.
- The same universal law of gravity applies to all of them.
- The law helps us to understand the planetary orbits, movements of such celestial bodies and how everything on each other.
Read More: Weightlessness
Important QuestionsQues: What is the gravitational force of attraction between the earth and a man whose weight is 70 kg who is standing at a sea level at a distance 6.38 x 106m. Ans: Mass of the earth m1 = 5.98x 1024 kg m2 = 70kg d = 6.38 x 106m G = 6.673 x 10-11 Nm2/kg2 Substituting in the equation \(F= G \frac{m_1m_2}{r^2}\) F = 685 N Ques: What is the force of gravity all over the surface of the earth? Ans: The force of gravity is not the same at different points on the earth surface. It is stronger at places with more underground mass than with places with a lower mass. The variation in the earth’s gravity is measured by NASA using two spacecrafts which are part of the Gravity Recovery and Climate Experiment (GRACE) mission. Ques: Why doesn’t the moon crash on the earth. Ans: The moon stays constant in its orbit around the earth due to the forces of speed and gravity. This makes moon hover around in the sky staying unaffected by gravity. Read More: Why doesn’t Moon Fall on the Earth |
Handwritten Notes on Gravitation
[Click Here for Sample Questions]
Some important handwritten notes on important concepts covered in gravitation are provided below.













Things to Remember
[Click Here for Sample Questions]
- Gravitation is the omnipresent universal force that attracts a body towards the centre of the Earth or any other physical body having mass.
- It is the weakest of all four fundamental forces present in nature.
- Universal equation of gravitation: F= Gm1m2/ r2
- The universal gravitational constant is the gravitational force of attraction between any two-unit masses separated by unit distance.
- The gravitational constant is denoted by ‘G’ and its value is 6.674×10−11 m3⋅kg−1⋅s−2.
Check Out:
Previous Year Questions
- What is the ratio of escape velocity of two planets? [BITSAT 2010]
- Calculate the speed of each particles with equal masses. [VITEEE 2013]
- Find the distance of the point from the moon? [JCECE 2007]
- Determine the relation petween the period and density of the artificial satellite? [JIPMER]
- Calculate the relative velocity of approach of two bodies of different masses. [AP EAPCET]
- What is the new time period of the satellite if its distance from the earth is increased? [BITSAT 2019]
- Calculate the work done to take a mass from point A to B. [BITSAT 2013]
- Calculate the new duration of earth day if the earth shrinks in density. [AP EAPCET]
- Calculate the speed of four identical particles. [JEE Main 2021]
- Calculate the acceleration due to gravity at 32 kms from earth surface. [JKCET 2004]
- Calculate the acceleration due to gravity below the earth’s surface. [AP EAPCET]
- What is the gravitational force on a body at a height half the radius of the earth from its surface. [NEET 2020]
- What is the escape velocity of an object projected at an angle of 450. [NEET 1993]
Sample Questions
Ques: What is gravitation? (1 Mark)
Ans: Gravitation or gravity is a force by which a is attracted towards the centre of the earth or any other physical body having mass.
Ques: What is the speed of gravity on Earth? (1 Mark)
Ans: Gravity is measured by the acceleration it gives to free-falling objects. At Earth’s surface, the acceleration of gravity is calculated as 9.8 meters per second.
Ques: What is Kepler's law of periods? Provide mathematical expression. (2 Marks)
Ans: It states that the square of the period of revolution of a planet around the sun is proportional to the cube of the semi-major axis of the elliptical orbit.
i.e. T2 ∝ R3
T2 = KR3
Ques: What is Newton’s law of gravitation? (2 Marks)
Ans: Every particle of matter in the universe attracts any other with a gravitational force varying directly as the product of the masses and inversely as the square of the distance between them. It can be mathematically written as,
F= GM1M2/ R2
Ques: What will be the gravitational force of attraction between the Earth and a 50 kg man standing at sea level, a distance of 6.38 x 106 m from the earth’s centre? (2 Marks)
Ans: Let us assume,
m1 is the mass of the Earth =5.98 x 1024 kg
m2 is the mass of the man = 50 kg
d = 6.38 x 106 m
G = 6.673 x 10-11 N m2/kg2
Now using the Gravitational force formula, we get at
F= (6.673x 10-11)(5.98X 1024)(50)/ (6.38X10-11)2 N=490.17 N
So, the gravitational force (F) will be 490.17N.
Ques: Assume that a geostationary Satellite Orbits the Earth at a Height of Nearly 36,000km from the surface of the Earth. What Is the Gravitational Potential Due to Earth's Gravity at the Satellite? (take the Potential Energy at Infinity = 0; Mass of the earth = 6.0×1024kg; radius =6400km.) (3 Marks)
Ans: Mass of the Earth, M=6.0×1024kg
The radius of the Earth, R=6400km=6.4×106m
Height of the satellite from the Earth’s surface (h)=36000km=3.6×107m
Gravitational potential energy due to Earth’s gravity at that height
PE=−GM(R+h) =(−6.67×10−11×6.0×1024)/(3.6×107+0.64×107)= −9.4×106Jk
So, the potential energy will be −9.4×106Jk.
Ques: Provide an Expression Showing Variation of Acceleration Due to Gravity with Height. (3 Marks)
Ans: Acceleration due to gravity at the earth’s surface is
g=GM/R2......(i)
[G = gravitational constant, g = gravity, R = Radius and M=mass of the earth]
If gh is the acceleration due to gravity at a point at the height of ′h’ above the surface of the earth,
gh=GM/(R+h)2 ......(ii)
Now dividing (ii) by (i), we get,
gh/g=GM/(R+h)2 . R2/GM
or,
gh=g(R2/(R+h)2)
If h<<,then the above relation becomes
gh = g(1+ h/R)−2
gh=g(1−2h/R)
(Expanding binomials and neglecting higher power)
So, the expression of gravity at height of ‘h’ will be gh=g(1−2h/R) .
Ques: Mention conditions under which the weight of a person can become Zero. (3 Marks)
Ans: (i) When the person is at the centre of the earth.
(ii) When the person is at the null points in space (at these points the gravitational forces due to different masses cancel each other)
(iii) when a person is standing in a freely falling lift.
(iv) When a person is inside a spacecraft that is orbiting around the earth.
Also Check Out:





Comments