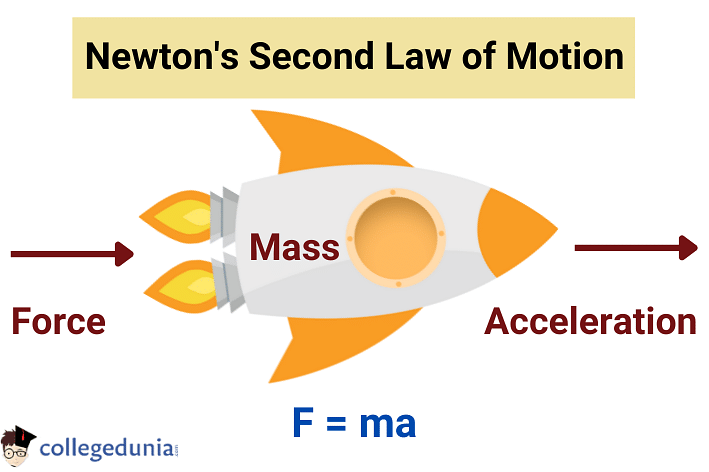
Newton's Second Law of Motion: Derivation & Applications

Content Writer

Newton’s Second Law of Motion is one of the three fundamental laws of motion proposed by Sir Isaac Newton. Newton’s Second Law of Motion states that the rate of change of momentum of an object is proportional to the applied force.

Newton’s second law of motion states the relationship between the force and acceleration of an object.
According to the law, an object will not change its motion unless a force acts on it.
Newton’s second law of motion quantitatively describes the changes a force produces in the motion of the body.
The law states that the rate of change of momentum of an object is equal to the applied force in magnitude and direction.

Mathematically, Newton’s Second Law of Motion is expressed as

\(Force ∝ {Change \;in \;Momentum \over Time \;Taken}\)

Table of Content

Newton's Second Law of Motion
What is Momentum?
What is Net Force?
Derivation of Newton’s Second Law of Motion
Applications of Newton’s Second Law of Motion
Things to Remember
Previous Years’ Questions
Sample Questions

Key Terms: Newton’s Second Law of Motion, Motion, Acceleration, Force, Momentum, Unbalanced Force, Magnitude, Velocity, Mass

Newton’s Second Law of Motion

[Click Here for Sample Questions]

Newton’s Second Law of Motion gives the relationship between the force and acceleration of any object in the universe. The law states that

“The rate of change of momentum of an object is directly proportional to the applied force.”

According to the Newton’s Second Law of Motion:

Force is equal to the rate of change of momentum in an object.
For a constant mass, force is equal to the product of mass and acceleration.

Laws of Motion Video Lecture

Acceleration is directly proportional to the unbalanced force and inversely proportional to the mass of the object.
If the force acting upon an object is increased, the acceleration of the object will also be increased.
If the mass of an object is increased, the acceleration of the object will be decreased.

Newton's Second Law of Motion

Newton’s Second Law of motion is mathematically expressed as

\(Force ∝ {Change \;in \;Momentum \over Time \;Taken}\)

Using the appropriate symbols, it can be written as

\(F ∝ {dP \over dT}\)

Where

F is the Force.
dP is Change in Momentum.
dT is Change in Time Taken.

Read More:

Relevant Concepts
Force and Laws of Motion	Newton's First Law of Motion	Newton's Laws of Motion
Newton's Third Law of Motion	Equations of Motion	Force and Motion

What is Momentum?

[Click Here for Previous Years' Questions]

Momentum of a body is defined as the product of the mass of the body and its associated velocity.

Momentum is a vector quantity which means it has both magnitude as well as direction.
According to the second Law of motion, the time rate of change of momentum is equal to the force acting on the particle.
Mathematically, Momentum = Mass × Velocity.
The unit of momentum is kg m/s.

Momentum (p) of an object of mass (m) moving with velocity (v) is given as:

p = m × v

What is Net Force?

[Click Here for Sample Questions]

Net force is the sum of all forces acting on a body or an object.

Net force is the vector sum of all forces acting on a body.
It is denoted by the symbol ΣF.
Net force leads to the acceleration of the mass.

Example: Consider that two forces of the magnitude of 30 N and 20 N are exerted on the right and left of the horse. Assuming the rightward direction as positive, the net force on the horse will be calculated as:

ΣF = 30 N – 20 N = 10 N
ΣF = 10 N to the right

Net Force

Read More: Laws of Motion MCQs

Derivation of Newton’s Second Law of Motion

[Click Here for Previous Years' Questions]

Newton’s second law of motion states that

\(Force ∝ {Change \;in \;Momentum \over Time \;Taken}\)

Now,

Initial momentum p₁= mu
Final momentum p₂ = mv

Therefore,

Change in Momentum = p₂ – p₁ = mv – mu = m(v – u)

Substituting the values in the equation, we get

\(F ∝ {m(v-u) \over t}\)

\(F ∝ {ma } \;\;\;[{(v-u) \over t} = a ] \)

\(F = kma\)

Here,

F is Force.
m is Mass of the Object.
u is Initial Velocity.
v is Final Velocity.
a is Acceleration

k is the constant of proportionality and is equal to 1 when the values are taken in the SI unit. Hence, Newton’s Second Law of Motion is expressed as

F = ma

Read More: Mathematical Formulation of Second Law of Motion

For Changing Mass

Assume a body to be at an initial point (0) at location L₀and at the time instance t₀.

Let us assume the body has mass m₀and travels with a uniform velocity of v₀.
Force F is applied to the body such that the body moves to point 1, situated at the location L_1, and reaches the point at time t₁.
The mass and velocity of the body undergo a transformation as the body travels to v₁.

Second Law of Motion for Changing Mass

On deriving the values for m₁and v₁, we get

\(F = {m_1v_1-m_0v_0 \over t_1-t_0}\)

For Constant Mass

For a constant mass, Newton’s second law can be equated as follows:

\(F = m{v_1-v_0 \over t_1-t_0}\)

Read More: Mass Formula

Solved Examples on Newton’s Second Law of Motion

Example 1: What will be the net force required in order to accelerate a 1000 kg car at 4 m/s²? (2 marks)

Solution: Given that

Mass of Car (m) = 1000 kg
Acceleration of Car (a) = 4 m/s²

Using Newton’s Second Law of Motion,

F = m × a

1000 kg × 4 m/s² = 4000 N

Thus, the horizontal net force which is required to accelerate 1000 kg is 4000 N.

Example 2: What will be the acceleration if the net force of 12 N is applied to a 1 kg object?

Solution: It is given that,

Force applied on Object (F) = 12 N.
Mass of Object (m) = 1 kg

Using Newton’s Second Law of Motion,

F = m × a

a = F / m
a = 12 N / 1 kg
a = 12 m/s²

Thus, the acceleration of the object will be 12 m/s².

Applications of Newton’s Second Law of Motion

[Click Here for Sample Questions]

Newton’s second law of motion is used in identifying the amount of force needed to make an object move or to make it stop. Given below are a few examples and phenomena for which the explanation is provided by Newton’s second law of motion.

Pushing a Cart

Force is directly proportional to the mass of the object.
Thus, we can see that it is easier to push an empty cart in a supermarket than it is to push a loaded one.
A heavier mass upon application of the same force will show a smaller acceleration.

Applications of Newton's Second Law of Motion

Kicking a Ball

In order to kick a ball, we exert force in a specific direction.
The stronger the force we put on it, the stronger the ball is kicked, and the further away it will travel.

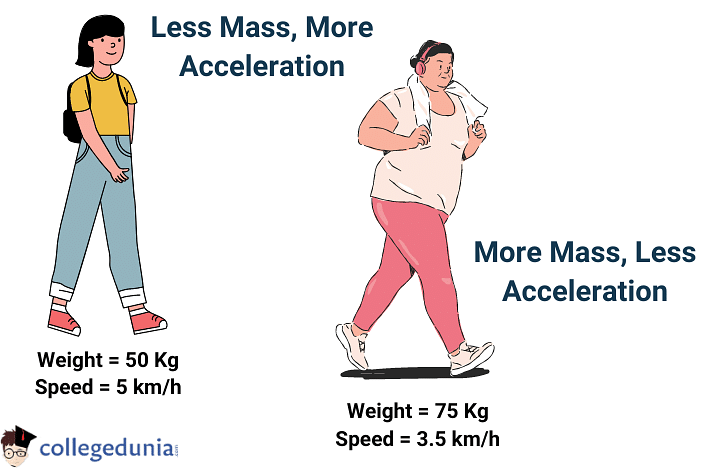
Two People Walking

Consider two people walking on a road.
if one person is heavier than the other, the one weighing heavier will walk slower
It is because the acceleration of the person weighing a lighter is greater.

Applications of Newton's Second Law of Motion

Also Check:

Related Topics
Motion in a Straight Line	Types of Motion Measurement	Uniform Motion and Non-Uniform Motion
Uniform Circular Motion	Acceleration Formula	Gravitational Acceleration Formula

Things to Remember

Newton’s Second Law of Motion states that the rate of change of momentum of an object is proportional to the applied force.
Acceleration is directly proportional to the unbalanced force and inversely proportional to the mass of the object.
Newton’s Second Law of Motion is expressed as Force ∝ Change in Momentum / Change in the Time Taken.
According to the law, F = ma, where m is the mass of the object and a is the acceleration.
Newton’s second law of motion is applicable when there is an unbalanced force acting on an object.

Previous Years’ Questions

From the top of a tower, a body A is projected vertically up … [JKCET 2007]
Assuming earth to be an inertial frame … [JKCET 2009]
A body sliding on a smooth inclined plane requires … [JIPMER 1999]
A body of mass 5 kg is suspended by a spring balance on an inclined plane … [BITSAT 2006]
A body of mass M hits normally a rigid wall with velocity V … [BITSAT 2018]
A man of weight 80 kg is standing in an elevator … [DUET 2003]
A light inextensible string that goes over a smooth fixed pulley … [BITSAT 2013]
A ball is released from the top of a tower … [JKCET 2007]
There are two identical springs each of spring constant … [UPSEEE 2017]
A 10gm bullet moving directly upward at 1000 m/s strikes … [UPSEEE 2017]
A body slides down a frictionless inclined plane starting from rest … [UPSEE]
A force F = 75N is applied on a block of mass 5kg … [UPSEE 2016]
A wooden box lying at rest on an inclined surface of a wet wood … [TS EAMCET 2017]
Two forces P and Q of magnitude 2F and 3F … [JEE Mains 2019]

Sample Questions

Ques. What is Newton’s Second Law of Motion? (3 Marks)

Ans. Newton’s Second Law of Motion is one of the three Laws of Motion given by Isaac Newton. Newton’s Second Law of Motion states that force is equal to the rate of change of momentum. It states that the acceleration of an object is directly proportional to the magnitude of the net force, in the same direction, and inversely proportional to the mass of the object. According to the law, F = ma, where m is the mass of the object and a is the acceleration.

Ques. What will be the net force needed to accelerate a 2500 kg truck at 5.50 m/s²? (3 Marks)

Ans. Given that

Acceleration (a) = 5.50 m/s²

Mass of the Truck (m)= 2500 kg

Using Newton’s Second Law of Motion

Force = mass × acceleration

F = 2500 × 5.5

F = 13750 N

Thus, the net force needed to accelerate a 2500 kg truck at 5.50 m/s² is 13750 N.

Ques. Calculate the acceleration if a net force of 6 N is applied on 0.5 kg-material. (3 Marks)

Ans. We are given that,

Force (F) = 6 N

Mass (m) = 0.5 kg

Acceleration (a) =?

Using Newton’s Second Law of Motion

Force = mass × acceleration

F = m × a

a = F/m

a = 6/0.5

a = 12 m/s²

Thus, the acceleration of the material is 12 m/s².

Ques. Give some real-life applications of Newton’s Second Law of Motion. (3 Marks)

Ans. Some of the applications of Newton’s Second Law of Motion in real life are:

It is easier to push a car in comparison to a truck with the same amount of force as the mass of the car is lesser than the mass of the truck.
In a golf game, the change in acceleration of the golf ball is directly proportional to the force with which it is being hit by the player through the golf stick.
Rockets tend to move faster due to the force applied known as thrust and undergo a change in acceleration.

Ques. A net force of 70 N is constantly applied on a 1.4 kg body at rest. Calculate the time taken by the body to attain the velocity of 150 m/s. (3 Marks)

Ans. Given that,

Force (F) = 70 N
Mass of Body (m) = 1.4 kg
Initial velocity = 0 m/s
Final velocity = 150 m/s
t = ?

First, we will calculate the acceleration using Newton’s Second Law of Motion,

Force = Mass × Acceleration

F = m × a

a = F/m
a = 70/1.4
a = 50 m/s²

Using the equations of motion, we get

v = u + at

150 = 0 + (50 × t)

150 = 50 × t

t = 150/50 = 3 seconds.

Thus, the time taken to attain the velocity of 150 m/s is 3 seconds.

Ques. What is Motion? (2 Marks)

Ans. Motion is defined as the change in position of a body based on its surroundings in a given time interval. The motion of any object with mass can be measured in distance, displacement, speed and velocity, acceleration, and time.

Ques. How is Newton’s second law applicable to a car crash? (2 Marks)

Ans. Newton’s second law of motion states that force is the product of mass and acceleration. The force in a car crash depends either on the mass or the acceleration of the car. If the acceleration or mass of the car increases, the force with which a car crash takes place will also increase, leading to a greater accident.

Ques. A body of mass 10 kg is moving with a constant velocity of 4 m/s on the frictionless ground. Find the force required to keep the body moving with the same velocity. (3 Marks)

Ans. It is given that

Mass (m) = 10 kg

Acceleration (a) = 0 (As the velocity is the same.)

F =?

Using Newton’s Second Law of Motion,

Force = mass × acceleration

F = 10 × 0

F = 0

Ques. How is Newton’s second law of motion applicable to rockets? (3 Marks)

Ans. According to Newton’s second law of motion, force is a product of mass and acceleration (F = ma). When force is applied to a rocket, it is called thrust. The greater the thrust or force will be, the greater will be the acceleration of the rocket. Acceleration is also dependent on the mass of the rocket’. It means the lighter the rocket, the faster will be the acceleration or vice versa.

Ques. Calculate the net force required to accelerate a 1000 kg car at 8 m/s². (3 Marks)

Ans. It is given that

Acceleration of Car (a) = 8 m/s²

Mass ofCar (m)= 1000 kg

Using Newton’s Second Law of Motion,

F = m × a

F = 1000 kg × 8 m/s²

F = 8000 N

Check-Out:

Class 11 & 12 PCMB Study Guides
NCERT Solutions for Class 11 Physics	NCERT Class 11 Physics Book	Important Derivations in Physics
Physics Study Notes	Formulas in Physics	Important Chemistry Formulas
Topics for Comparison in Physics	NCERT Solutions for Class 6 to 12	Topics with relation in physics
NCERT Solutions for Class 12 Biology	Class 12 Physics Book PDF	Class 12 Physics Practicals
Biology MCQs	SI units in Physics	Important Physics Constants and Units
Physics MCQs	Chemistry Study Notes	Comparison topics in Chemistry
Comparison Topics in Biology	NCERT Solutions for Class 12 Maths	Class 12 Physics Notes
Biology Study Notes	Class 12 Biology Notes	Class 12 Maths Notes
Class 12 PCMB Notes	NCERT Class 12 Biology Book	NCERT Class 12 Maths Book
Chemistry MCQs	NCERT Solutions for Class 11 Maths	NCERT Solutions for Class 11 Biology
NCERT Solutions for Class 11 Chemistry	NCERT Solutions for Class 12 Chemistry	Class 12 Chemistry Practicals
Maths MCQs	NCERT Class 11 Biology Book	Class 12 Chemistry Notes
Class 11 PCMB Syllabus	Important Maths Formulas	NCERT Solutions for Class 12 Physics

Newton's Second Law of Motion: Derivation & Applications