
Content Writer-SME | Updated On - Nov 15, 2024
The energy of orbitals is determined by the energy of electrons present in them. Electrons have their own energy and are negatively charged particles. An electron's energy the orbit number of an atom. Each orbital has a three-dimensional shape and distinct energy. The electrons surrounding the nucleus are assigned to orbitals in quantum theory, which are not to be confused with the orbits of the solar system.
Due to the unusual behavior patterns of atoms and molecules, the study of chemistry progressed through the ages, resulting in the creation of Mendeleev's periodic table and Rutherford's atomic model, which was later corrected by Bohr. We will study Energy of orbitals, their principles, factors that affect them, and much more in this article.
Read Also: Discovery of Electron
| Table of Content |
Key Terms: Orbital Energy, Energy Level Diagram, Principal Quantum Number, Azimuthal Quantum Number
What is Energy of Orbitals?
[Click Here for Sample Questions]
The energy necessary to take an electron present in an orbital to infinity, or the energy released when an electron from infinity is added to that orbital, is referred to as orbital energy or energy of orbital. This orbital energy is determined by the principal quantum number (n) and the azimuthal quantum number (l), both of which are determined by the shell and subshells. All orbitals belonging to the same subshell have the same energy, and orbitals having the same energy are referred to as degenerate orbitals.
The principal quantum number alone can indicate the energy of an electron in a single atom. The following is a list of orbitals in ascending order of orbital energy:
1s<2s = 2p<3s = 3p = 3d<4s = 4p = 4d = 4f

Orbital Energy
Principal Quantum Number (n)
Bohr demonstrated that electron orbitals indicate an energy level in terms of their distance from the nucleus, which laid the groundwork for orbital chemistry. The principal quantum number (symbolized n) is one of four quantum numbers allocated to each electron in an atom to characterize the state of that electron in quantum mechanics. It is a discrete variable (starting from 1) as its values are natural numbers. In ascending order, the Orbitals are designated K, L, M, N... or 1, 2, 3, 4... These numbers are Principal Quantum Numbers. The letter 'n' stands for a Principal Quantum Number. For example, n = 1 for the K-orbital, n = 2 for the L-orbital, and n = 3 for the M-orbital.

Principal Quantum Number and Azimuthal Quantum Number
Read More:
| Related Articles | ||
|---|---|---|
| Electron Spin | Pauli Exclusion Principle | |
Azimuthal Quantum Number (l)
Arnold Sommerfeld looked into the chemistry of orbitals and discovered that each orbital energy level or shell is made up of several subshells. He believed that, in addition to the circular orbits established by Bohr, there are also elliptical orbits. The Azimuthal or Subsidiary quantum number aids in determining the subshells' ellipticity. The letter 'l' is commonly used to represent it.
An azimuthal quantum number is a quantum number that characterizes the form of an atomic orbital and defines its orbital angular momentum. The azimuthal quantum number is second in a series of quantum numbers that describe an electron's singular quantum state. It's also known as the second quantum number, orbital quantum number, or orbital angular momentum quantum number.
Some spectroscopic symbols are used to express the “l” value in place of 1,2,3.. which are as follows-
| ‘l’ Value | Spectroscopic Symbol |
|---|---|
| 0 | s |
| 1 | p |
| 2 | d |
| 3 | f |
| 4 | g |
Check Out: Quantum Theory of Light
How to Calculate Energy Level of Orbitals?
[Click Here for Sample Questions]
The orbital energy, or the energy of a single electron in a hydrogen-like atom, is solely determined by the primary quantum number (n). When it comes to populating the atom with electrons, the Aufbau principle dictates that the lower energy level orbitals always come first in orbitals chemistry. The following order is used as follows: 1s < 2s < 2p < 3s < 3p < 4s... and so on.
- The electrons repel each other because they are negatively charged particles. The attraction between electrons, the positively charged nucleus, and the repulsive force within the electrons all contribute to an atom's stability. Only if the total attractive interaction exceeds the total repulsive interaction can the particle remain stable.
- The atomic number increases as we proceed down the periodic table, and another factor, shielding, comes into the scene. The overall positive charge exerted by the Nucleus (Ze) is slightly hindered for the electrons in the outer shells due to the existence of electrons in the inner shells. An effective nuclear charge (Zeffe) is the net positive charge felt by electrons in the outer shells.
- The orbital would be more closely linked, the closer it gets to the nucleus. As a result, an s-orbital electron will be stronger than a p-orbital electron in its bonding to the atom's nucleus.
- The s-orbital particles will have a lower charge due to their lower orbital energy, implying that they will be more negative than the p-orbital electrons, which will have lower energy due to their greater orbital energy compared to the d-orbital electrons.
- When two orbitals have the same n+l value, the orbital with the lowest n (principal quantum number) count has the lowest energy.
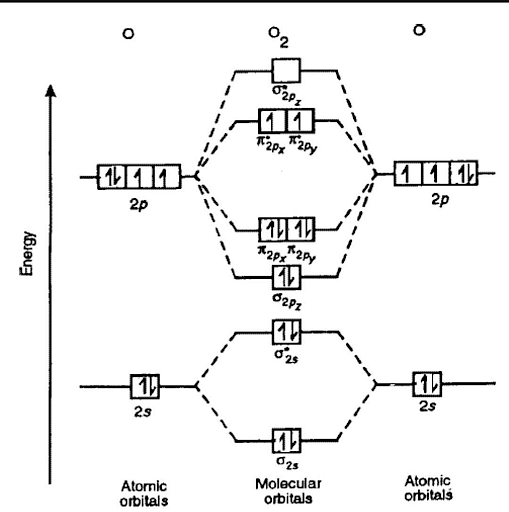
Orbital Energy Level Diagram for O2
Energy Level Diagrams: Some Important Observations
[Click Here for Sample Questions]
The energy level diagram can help us deduce that the energy of the subshells of a specific shell is not equal. The energies of 2s and 2p, for example, are different.
- The subshell with the lowest value of I has the lowest energy in a given shell. 2s (I = 0) has lower energy than 2p (I = 1) in the second shell. However, in shell 3, energy is arranged in the following order: 3s < 3p < 3d.
- The shape of the orbitals are represented by the letters s, p, d, and f. The Nucleus is in the center of the s-orbital, which is spherical. The p-orbital has a pair of lobes on each side of the Nucleus, resembling a dumbbell.
- With an increase in the value of n, the lower shell's subshell may have more energy than the upper shell's, therefore 3d has more energy than 4s.
- The differences between the energies of the s and p subshells are small for the same value of n, but they are substantial between the p and d subshells, and so on.

Electrons in Energy Level Diagram
Factors that Affect Orbital Energy
[Click Here for Sample Questions]
The orbital energy in the same subshell falls as the atomic number (Zeff) increases.
- The s orbital electron will be more strongly coupled to the nucleus for a given value of the primary quantum number than the p orbital electron, which will be more tightly bound in contrast to a d orbital electron.
- The energy of s orbital electrons is more negative or less than that of p orbital electrons. The p orbital electrons will have less energy than the d orbital electrons in this situation.
- Unlike multi-electron atoms, which are dictated by both the main quantum number and the azimuthal quantum number, the energies of orbitals of hydrogen and hydrogen-like particles are exclusively determined by the principal quantum number.
- Because the shielding from the nucleus varies for electrons in different orbitals, energy levels with the same primary quantum number are divided. As a result, the orbital energy would be determined by the values of the primary quantum number and the azimuthal quantum number, both denoted by the letters n and l. As a result, an orbital's energy decreases as (n + 1) decreases.
Things to Remember
- Energy level diagrams are diagrams that show the arrangement of orbitals in order of increasing energy.
- The principal quantum number alone can indicate the energy of an electron in a single atom. In multi-electron atoms, an electron's energy is determined by both its principal quantum number (n) and its azimuthal quantum number (l).
- The mutual repulsion among the electrons in a multi-electron atom is primarily responsible for the difference in energy of multiple subshells dwelling in the same shell.
- The presence of electrons in the inner shells prevents electrons in the outer shell from experiencing the full positive charge of the nucleus in larger atoms (Ze).
- The outer shell electrons are shielded from the nucleus by the inner shell electrons, which is known as the shielding effect.
- The effective nuclear charge (Zeffe) is the net positive charge experienced by outer shell electrons.
Read More: Electric Charges and Fields
Sample Questions
Ques. Which orbital has a higher degree of orbital energy, 3d or 4p? (2 marks)
Ans. The 3d orbital's (n+l) value is (3+2) = 5, while the 4p orbital's (n+l) value is (4+1)=5. Both have the same (n+l) value, but 3d has a smaller n-count and consequently a lower orbital energy level. The answer will be 3d.
Ques. What is the smallest value of n that allows for the existence of g orbitals? (1 mark)
Ans. l = 4 for g-orbitals.
The Azimuthal quantum number (l) can have a value of zero to (n – 1) for any value 'n' of the principal quantum number. So, the minimum value of n = 5 for l = 4.
Ques. Which orbital has the lowest orbital energy level, 3d or 4s? (2 marks)
Ans. The 3d orbital's n+l value is (3 + 2) = 5, whereas the 4s orbital's (n + l) value is (4 + 0) = 5. As a result of the greater (n+l) value, the 4s orbital has a higher orbital energy level. So the answer will be 3d.
Ques. The quantum numbers +1/2 and -1/2 for an electron denote two quantum mechanical spin states with no classical analogs. Please explain. (3 marks)
Ans. The assertion here implies that electron spin quantum states +1/2 and -1/2 exist. The spin quantum number is a property that is conserved. The spin of an electron is described by these two states +1/2 and -1/2. It is a term used only in quantum physics to describe the property of an electron, and it has no confirmation in classical physics. It is for this reason that the term "no classical analogue" is applied.
Ques. Why is the s-energy orbital lower than the d- orbital's? (2 marks)
Ans. The s-orbital particles will have a lower charge due to their lower orbital energy, implying that they will be more negative than the p-orbital electrons, which will have lower energy due to their greater orbital energy compared to the d-orbital electrons.
Ques. What is the order in which the orbital energy increases? (2 marks)
Ans. 1s<2s = 2p<3s = 3p = 3d < 4s = 4p = 4d = 4f is the order in which the energy of various orbitals increases. In multi-electron atoms, however, the energy of an electron is determined by both its main quantum number (n) and its azimuthal quantum number (l).
Ques. What causes the energy of an orbital to be lower? (2 marks)
Ans: The energy of an orbital decrease as the value of (n + l) decreases. When two orbitals with the same (n + l) value have the same energy, the orbital with the smaller n (principal quantum number) has the lower energy.
Ques. For n+l = 4, what is the greatest number of electrons with the same spin that can be found in an atom? (5 marks)
Ans. Given that n+l = 4, so we have to find out the possible values of n and l whose sum will be 4. Hence, the possible values will be n=3 and l=1 & n=4 and l=0.
For the values of n and l be 3 and 1 respectively-
3p subshell is represented by n=3 and l=1. So, the maximum number of electrons in this subshell will be 6.
For the values of n and l be 4 and 0 respectively-
4s subshell is represented by n=4 and l=0. So, the maximum number of electrons in this subshell will be 2.
As, the total number of electrons will be = 6+2= 8, so the greatest number of electrons with the same spin will be = 8/2= 4.
Check-Out:





Comments