
Content Writer
Center of Mass is a position on an object that represents the average mass of that object.
- It is the mean location of a distribution of mass in space.
- The center of mass of a rigid body with uniform density is located at the centroid.
- For instance, the center of mass of a circular plate would be at its center.
- In some cases, the center of mass doesn't fall anywhere on the object.
- For instance, the center of mass of a ring is located at its center, where there is no material.
It is the unique position where all the weighted position vectors of all the parts of a system add up to zero. Centre of Mass helps to analyze the complicated motion of the system of objects, particularly when two and more objects collide or an object breaks down into fragments.
Read More: NCERT Solutions For Class 11 Physics System of Particles & Rotational Motion
Key Terms: Center of Mass, Center of Gravity, Center of Mass Formula, Vectors, Mass, Gravity, System of Particles, Table Edge Method
What is Center of Mass?
[Click Here for Sample Questions]
Center of Mass is defined as a point where the entire mass of the body or all masses of a system of particles appears to be concentrated.
- It is a point at the center of the distribution of mass in space where the weighted relative position of the distributed mass has a sum equal to zero.
- It is also known as the point of equilibrium.
- In simpler terms, the center of mass is a position relative to an object.
- It is the average position of all parts of the system or the average position of a mass distribution in space.
- It is a point at which a force is usually applied resulting in linear acceleration without any angular acceleration.

Center of Mass
Read More:
Center of Gravity
[Click Here for Previous Years’ Questions]
Centre of Gravity is defined as a point through which the force of gravity acts on an object.
- It is the point where the resultant torque due to gravity forces disappears.
- In most mechanics problems, the gravitational field is considered to be uniform, thus, the center of gravity and center of mass will be at the same position.
- Both these terms are used interchangeably since they are often at the same position or location.
Read More: Difference Between Centre of Gravity and Centroid
System of Particles
[Click Here for Sample Questions]
System of Particles is defined as a collection of a large number of particles that may or may not interact with each other or may be connected to each other.
- These particles can be real particles of rigid bodies in translatory motion.
- They are the particles that interact with a mutually applying force.
- The forces of mutual interaction between these particles are called the internal forces of the system.
- These internal forces exist in pairs of equal size and opposite directions.
- Apart from internal forces, external forces can also act on all or part of the particles.
- External force indicates a force acting on any particle, included in the system by another body external to the system.
Read More: System of Particles & Rotational Motion Important Questions
How to Determine Center of Mass?
[Click Here for Previous Years’ Questions]
In order to determine the center of mass of a body, the forces of gravity acting on the body need to be taken into consideration.
- It is considered because the center of mass is the same as the center of gravity in the parallel gravitational field near the earth's surface.
- In addition, the center of mass of a body with an axis of symmetry and constant density will lie on this axis.
- Likewise, the center of mass of a circular cylinder with constant density will have its center of mass on the axis of the cylinder.
- In a body with spherical symmetry of constant density, its COM is at the center of the sphere.
The two main methods to determine the center of mass of an object as described below:
Table Edge Method
- This method is used to find the center of mass of rigid objects with at least one flat side.
- The object is pushed slowly without rotating the surface of a table toward the edge.
- A line is drawn parallel to the edge of the table at the point where the object is just about to fall.
- The same procedure is repeated with the object being rotated 90°.
- The point of intersection of the two lines gives the center of mass.
Plumb Line Method
- This method is used for objects that can be suspended freely about a point of rotation.
- A piece of cardboard with an irregular shape suspended on a pinboard is an example of this method.
- The cardboard rotates freely around the pin under gravity and reaches a stable point at last.
- Then, a plumb line is hung from the pin and is used to mark a line on the object.
- The pin is then moved to another location and the procedure is repeated again.
- The center of mass then lies beneath the point of intersection of these two lines.
Read More: System of Particles and Rotational Motion MCQs
Center of Mass Formula
[Click Here for Sample Questions]
Center of mass is generally calculated by vector addition of the weighted position vectors which point to the center of mass of each object in a system. To avoid the use of vector arithmetic, we can calculate the center of mass separately for components along each axis.
Thus, the Center of Mass Formula is as follows:
\(x_{cm} = \frac{\Sigma^N_{i=1} m_i x_i}{M}\)
\(y_{cm} = \frac{\Sigma ^N_{i=1} m_iy_i}{M}\)
\(z_{cm} = \frac{\Sigma^N_{i = 1} m_iz_i}{M}\)
The above formulas are used in the case of pointed objects. If we need to find the center of mass of an extended object like a rod, we will consider the differential mass and its position and then integrate it over the entire length.
\(X_{com}=\frac{∫~x ~dm}{M}\)
\(Y_{com}=\frac{∫~y ~dm}{M} \)
\(Z_{com}=\frac{∫~z ~dm}{M} \)
Where
- Xcom, Ycom, Zcom: Center of Mass along x, y, and z-axis
- M: Total Mass of System
- n: Number of Objects
- mi: Mass of the ith Object
- xi: Distance of ith object from the x-axis
Read More: Important Formulas for System of Particles and Rotational Motion
Solved ExampleExample: The minute hand of a wall clock is made up of an arrow with a circle connected by a metal piece with almost zero mass. The mass of the circle is 60.0 g while the mass of the arrow is 15.0 g. Calculate the center of mass if the circle is at position 0.000 m and the arrow is at position 0.100 m. Solution: Given that
Using the Centre of Mass Formula, the center of mass of the minute hand will be \(x_{com} = \frac{\sum_{n}^{i=0}m_ix_i}{M}\) \(x = \frac{\sum_{n}^{i=0}m_ix_i}{M}\) \(x = \frac{m_1x_1+m_2x_2}{m_1+m_2}\) = \(\frac{60 \times 0.0 + 15 \times 0.10}{60 + 15}\) = \(\frac{1.50}{75}\) x = 0.02 m Thus, the center of mass of the minute hand will be at 0.020 m from the circle. |
Importance of Centre of Mass
[Click Here for Previous Years’ Questions]
Centre of Mass of a system is the point where any uniform force acts upon the object.
- Centre of Mass makes it simpler to solve the mechanics problems involving the motion of oddly-shaped objects and complicated systems.
- While doing calculations, it is assumed that the mass of an oddly-shaped object is concentrated in a tiny object at the Centre of Mass.
- This tiny object is sometimes referred to as the Point Mass.
- If a rigid object is pushed at its center of mass, then it will always move as if it is a point mass.
- Regardless of its shape, it will not rotate about any axis.
- The object will begin rotating about the center of mass if it is subjected to an unbalanced force at some other point.
Check More:
System of Particles and Rotational Motion Handwritten Notes
[Click Here for Sample Questions]
Here are the handwritten notes for System of Particles and Rotational Motion:















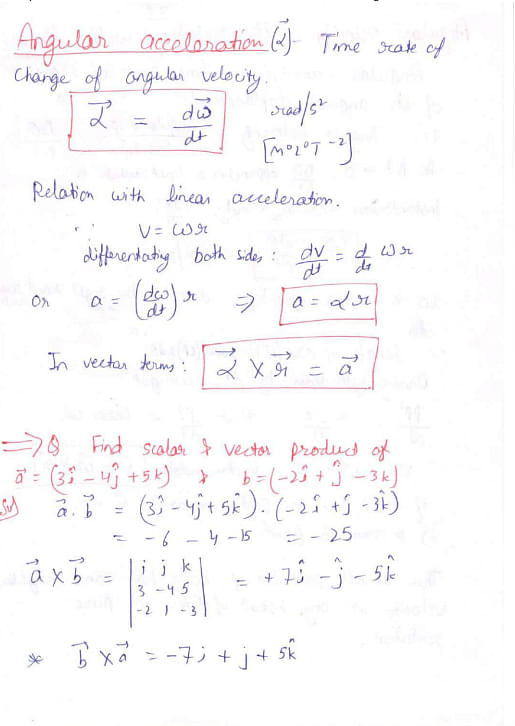






























Things to Remember
- Center of Mass is a position relative to an object or system of objects.
- It is the average position of the parts of the system, weighted respective to their masses.
- It is the point where all uniform forces on the object act together.
- Table Edge Method and Plumb Line Method are the two methods used to determine the center of mass.
- Center of Mass is calculated through vector addition of the weighted position vectors pointing to the center of mass.
- Centre of Gravity is an imaginary point at which the force of gravity appears to act.
Previous Years’ Questions
- Two blocks A and B each of mass m, are connected by… [JEE Advanced 1993]
- A solid sphere of mass 2kg… [AMUEEE 2007]
- A point mass of 1 kg collides… [JEE Advanced 2010]
- A quarter horse power motor runs at a speed of… [AMUEEE 2015]
- A shell is fired from a cannon with a velocity… [JEE Advanced 1996]
- A smooth sphere A is moving on a frictionless… [JEE Advanced 1999]
- A solid cylinder of mass M and radius R rolls… [JKCET 2017]
- A solid sphere is rolling down an inclined plane... [JKCET 2015]
- A thin uniform rod, pivoted at O, is rotating… [JEE Advanced 2012]
- A uniform bar of length 6 a and mass 8 m lies on… [JEE Advanced 1991]
Sample Questions
Ques. Define Center of Mass. (3 Marks)
Ans. Center of Mass is defined as a position on an object or a body that represents the mean mass of that object. For a simple object with a consistent geometric shape and mass, the center of mass is located at the central point of that object. For instance, a cuboid will have the center of mass at the center of that cuboid. In the case of objects without a consistent geometric shape, the center of mass is calculated using vectors and is located at the point where all the vectors of mass cancel each other out to zero.
Ques. Two point masses of 3 kg and 5 kg are located 4 m and 8 m from the origin on the X axis. Identify the position of the center of mass of the two point masses
(i) From the Origin.
(ii) From 3 kg of Mass. (5 Marks)
Ans. (i) From the Origin
The point masses are at positions, x1 = 4 m, x2 = 8 m from the origin along the X axis.

The center of mass xCM can be obtained using equation 5.4.
\(X_{CM} = \frac{m_1x_1 + m_2x_2}{m_1 + m_2}\)
\(X_{CM} = \frac{(3 \times 4) + (5 \times 8)}{3+5}\)
\(X_{CM} = \frac{12 + 40}{8} = \frac {52}{8} =6.5m\)
The center of mass is located 6.5 m from the origin on X-axis.
(ii) From 3 kg of Mass
The origin is shifted to 3 kg mass along the X-axis. The position of 3 kg point mass is zero (x1 = 0) and the position of 5 kg point mass is 4 m from the shifted origin (x2 = 4 m).

\(X_{CM} = \frac{(3 \times 0) + (5 \times 4)}{3 + 5}\)
\(X_{CM} = \frac{0 + 20}{8} =\frac {20}{8} =2.5 m\)
The center of mass is located 2.5 m from the 3 kg point mass, and 1.5 m from the 5 kg point mass on X-axis.
Ques. Locate the center of mass of a uniform rod of mass M and length l. (3 Marks)
Ans. Consider a uniform rod of mass M and length whose one end coincides with the origin as shown in Figure. The rod is kept along the x-axis. To find the center of mass of this rod, we choose an infinitesimally small mass dm of elemental length dx at a distance x from the origin.

\(\lambda\) is the linear mass density (i.e. mass per unit length) of the rod.
\(\lambda = \frac{M}{\ell}\)
The mass of a small element (dm) is,
dm = \(\frac{M}{\ell}\)dx
Now, we can write the center of the mass equation for this mass distribution as,
\(X_{CM} = \frac{\int xdm}{\int dm}\)
\(X_{CM} = \frac{\int^\ell_o \times (\frac{M}{\ell} dx)}{M} = \frac{1}{\ell} \int^\ell_o x dx\)
\(= \frac{1}{\ell}[\frac{x^2}{2}]^\ell_o = \frac{1}{\ell}(\frac{\ell^2}{2})\)
\(X_{CM} = \frac{\ell}{2}\)
As the position l/2 is the geometric center of the rod, it is concluded that the center of mass of the uniform rod is located at its geometric center itself.
Ques. Where is the center of mass of a two-particle system located? (1 Mark)
Ans. The center of mass in a two-particle system lies between the two masses on the line joining them and dividing the distance between them in the inverse ratio of their masses.
Ques. Can the geometrical center and center of mass of an object coincide? (2 Marks)
Ans. Yes, the geometrical center and center of mass of objects can coincide. Some of the objects whose geometrical center and center of mass coincide are
- Spheres
- Circular Rings
- Circular Discs
- Cubes
- Cylinders
Ques. What is the difference between the Center of Mass and the Center of Gravity? (2 Marks)
Ans. Center of Mass is the point at which the distribution of mass is equal in all directions. It is independent of the gravitational field. On the other hand, the center of gravity is the point where the weight distribution is equal in all directions. It is affected by the gravitational field. When the gravitational field is assumed to be uniform, the center of gravity is at the same position as the center of mass.
Ques. Calculate the center of mass if m1 = 2 kg and m2 = 5 kg are two-point Masses located at y1 = 10 m and y2 = -5 m respectively. (3 Marks)
Ans. Given that
- m1 = 2 kg
- m2 = 5 kg
- y1 = 10 m
- y2 = -5 m
Using the Center of Mass Formula, we get
ycom = (m1y1+m2y2+...)/(m1+m1+...)
ycom = (2×10+5×(−5))/(2+5)
ycom = (20−25)/7 = −5/7
Thus, ycom = −5/7
Ques. What is the Center of Mass Reference Frame? (3 Marks)
Ans. Reference Frame in Physics is used to refer to a coordinate system used for calculations. It has a set of axes ( x-axis and y-axis) and an origin (zero point). The reference frame is fixed relative to the laboratory and a convenient origin point is chosen. This type of frame is referred to as a laboratory reference frame.
Center of mass is used to define the origin of a moving reference frame for a system. It is thus called the COM frame. The COM frame is mainly used in collision problems. The momentum of a fully-defined system measured in the COM frame is always zero which means that calculations can often be much simpler when done in the COM frame compared to the laboratory reference frame.
Ques. What is Center of Gravity? (2 Marks)
Ans. Center of Gravity is the position of an object that defines the average weight of that object. It is the point where the action of gravity appears to pull down the object. The center of gravity is equal to the center of mass as long as the gravity is uniform across the entire area of the object. If the force of gravity is the same across the object, then the center of gravity would be in the geometric center.
Ques. How do find the Center of Mass? (3 Marks)
Ans. Center of mass can be found by the following steps:
- Take the masses you need to find the center of mass and multiply them by their positions.
- Add the obtained values together.
- Lastly, divide the value obtained by the sum of all the individual masses.
Check-Out:






Comments