
Content Writer
Average Velocity is the ratio of total displacement over total time. It is a measure of the average value of the known velocities.
- Velocity refers to the rate at which displacement changes in relation to time.
- Average Velocity is the total displacement traveled by the body in unit time.
- It is a vector quantity with both magnitude and direction.
- Average Velocity is denoted by the symbol ‘Vav’.
Average Velocity Formula is given as
| \({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\) |
Read More: NCERT Solutions For Class 11 Physics Motion in a Straight Line
| Table of Content |
Key Terms: Average Velocity, Average Velocity Formula, Velocity, Displacement, Average Speed, Vector, Initial Velocity, Time, Distance
What is Average Velocity?
[Click Here for Sample Questions]
Average Velocity is the rate of change in the position of an object in a given direction divided by the time taken.
- It is defined as the total displacement of the object divided by the total time taken.
- It is a vector quantity and is denoted by the symbol ‘Vav’.
- The SI unit of average velocity is meters per second (m/s).
Average Velocity Example: The velocity of an athlete running 4 km south may increase or decrease at certain times during the run. However, we can calculate the average velocity of the athlete by dividing the change in position (4 km) by the total time of the run.
Read More:
Average Velocity Formula
[Click Here for Sample Questions]
Average velocity is calculated by dividing the total displacement of the object divided by the total time taken. Thus, the Average Velocity Formula is given as:
| \({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\) |
(I) In case the initial and final distances xi and xf are given with their corresponding time intervals ti and tf, then the Average Velocity Formula is
| \(V_{av} = {X_f\,-\,X_i \over t_f\,-\,t_i}\) |
Where
- xi: Initial Distance
- xf: Final Distance
- ti: Initial Time
- tf: Final Time
(II) When Initial Velocity U and Final Velocity V are given, then, the Average Velocity Formula is
| \(V_{av} = {U\, + \, V \over2}\) |
Where
- U: Initial Velocity
- V: Final Velocity
(III) When different distances d1, d2, d3 ……. dn are given for different time intervals t1, t2, t3,… tn then, Average Velocity Formula is given as
| \(V_{av} = {d_1\, + \,d_2\,+\,d_3\,+\,.....\,d_n\,\over t_1\, + \,t_2\,+\,t_3\,+\,.....\,t_n}\) |
Read More: Motion in a Straight Line Important Questions
Solved Examples on Average Velocity Formula
[Click Here for Sample Questions]
Here are a few solved examples on the Average Velocity Formula to understand how to use the formula in various situations:
Example 1: A train moving with an initial velocity of 100 m/s reaches its destination at 150 m/s. What will be its average velocity?
Solution: Given that
Initial Velocity U = 100 m/s
Final velocity V = 150 m/s
Using the Average Velocity Formula,
\(V_{av} = {U\, + \, V \over2}\)
Vav = (100 + 150)/2
Vav = 250/2 = 125 m/s
Thus, the average velocity of the train is 125 m/s.
Example 2: The distance of a cycle between points A and B is 30 m, between B and C is 20 m, and between C and D is 15 m. What will be the average velocity between A and D, if the time taken in the given sections is 20 s, 10 s, and 5 s, respectively?
Solution: It is given that
- Distance between Points A and B = 30 m
- Distance between Points B and C = 20 m
- Distance between Points C and D = 15 m
- Time taken from Point A to B = 20 s
- Time taken from Point B to C = 10 s
- Time taken from Point C to D = 5 s
Using the Average Velocity Formula,
\(V_{av} = {d_1\, + \,d_2\,+\,d_3\,+\,.....\,d_n\,\over t_1\, + \,t_2\,+\,t_3\,+\,.....\,t_n}\)
Thus, by substituting the values, we get
\(V_{av} = {30\, + \,20\,+\,15\over 20\, + \,10\,+\,5}\)
\(V_{av} = {65\over 35} = 1.85\)
Thus, the average velocity of the cycle is 1.85 m/s.
Check More:
Difference Between Average Velocity and Average Speed
[Click Here for Sample Questions]
Average Speed and Average Velocity are expressed in the same units, however, they are totally different concepts in Physics. Average speed deals with the distance, while average velocity takes displacement into consideration. The difference between Average Speed and Average Velocity is given as follows:
| Average Speed | Average Velocity |
|---|---|
| Average Speed is the total distance traveled by an object divided by the total time taken. | Average Velocity is the total displacement divided by the total time taken. |
| Average Speed takes into account the total length of the path traveled. | Average Velocity takes into consideration the starting and ending points of the path covered by an object. |
| It is a scalar quantity with only magnitude. | It is a vector quantity with both direction and magnitude. |
| It is always positive. | It can be positive or negative depending on the direction. |
| Average Speed = Total Distance/Total Time Taken | Average Velocity = Total Displacement/Total Time Taken |
Motion in a Straight Line Class 11 Handwritten Notes
[Click Here for Sample Questions]
Here are the handwritten notes on Class 11 Motion in a Straight Line:




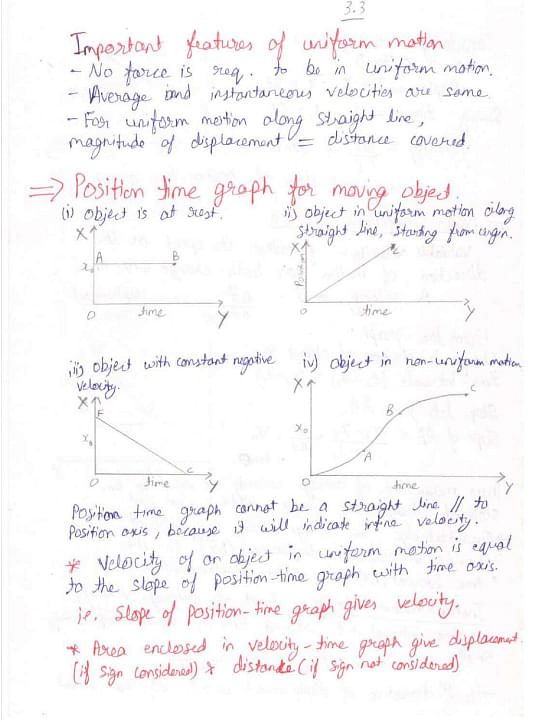
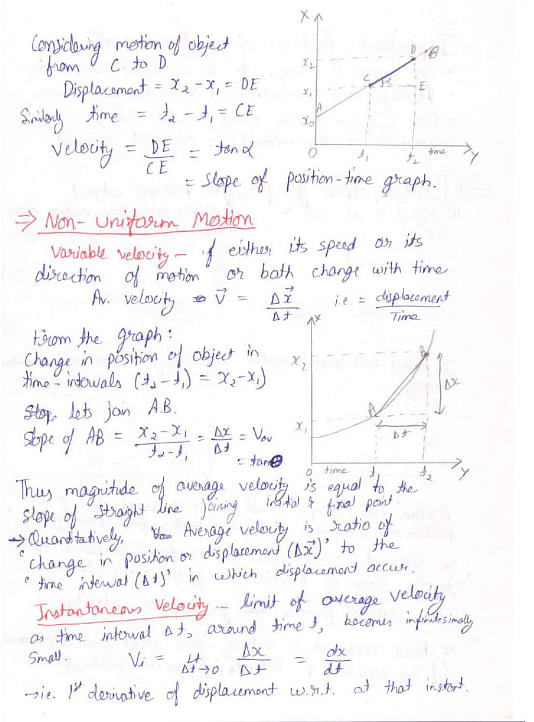



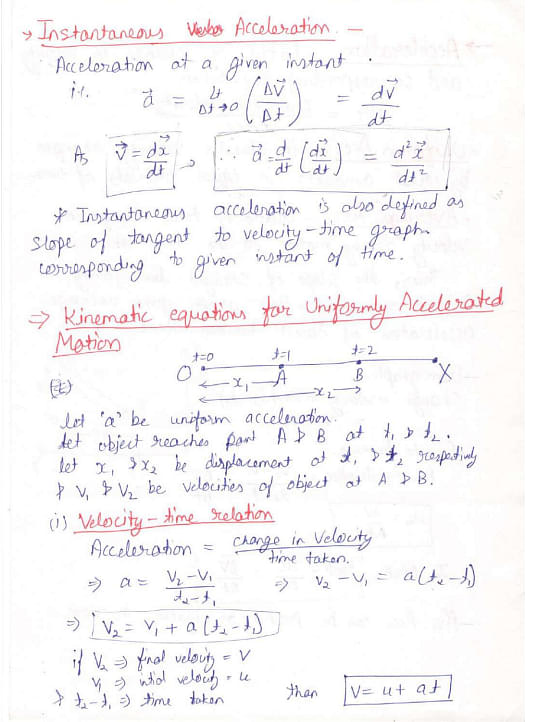

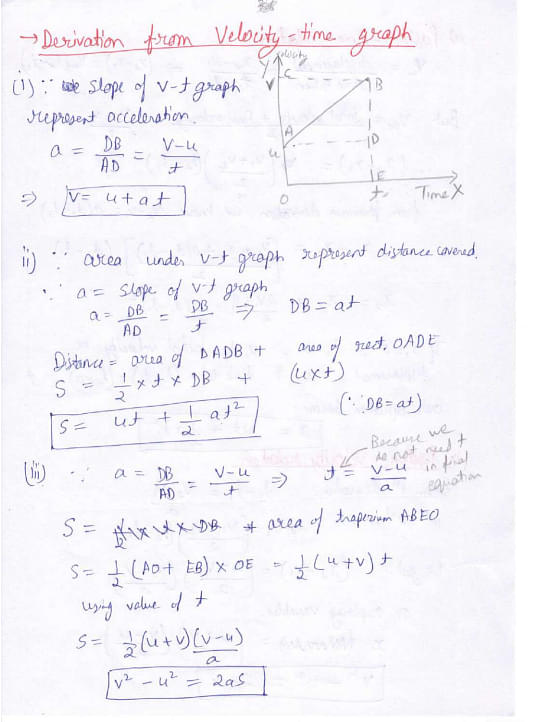




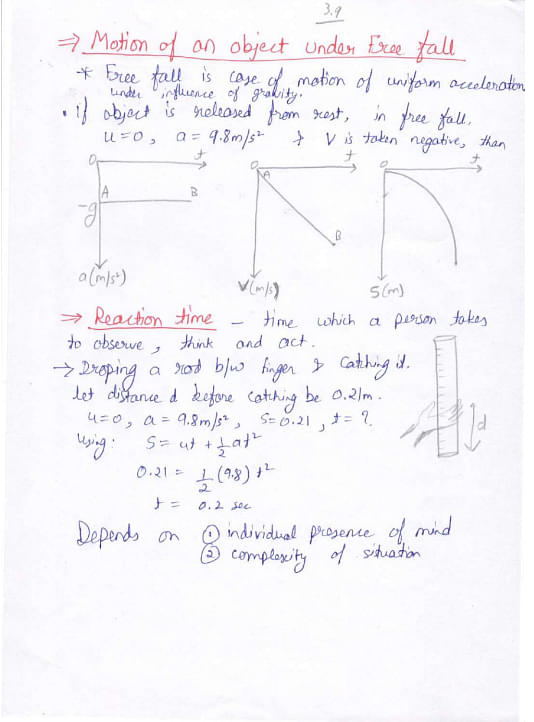
Things to Remember
- Average Velocity is the total displacement of an object in time t.
- Velocity is defined as the rate of change of an object’s position with respect to time.
- The SI unit of average velocity is m/s and it is a vector quantity.
- Average Velocity Formula is \({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\).
- It can also be calculated as \(V_{av} = {U\, + \, V \over2}\).
- Average Velocity is a function of displacement while Average Speed is a function of distance.
Sample Questions
Ques. The driver of the truck drives 20 m down the road in 5 minutes. He then backed away and drove 12 m back down the road in 3 minutes. What is the Average Velocity? (2 Marks)
Ans. Given that
- Initial Distance xi = 20 m
- Final Distance xf: = 12 m
- Initial Time ti = 5 sec
- Final Time tf = 3 sec
Using the Average Velocity Formula,
\(V_{av} = {X_f\,-\,X_i \over t_f\,-\,t_i}\)
Vav = (20 – 12) / (5+3)
Vav = 8/8
Vav = 1 m/s
Thus, the average velocity of the truck is 1 m/s.
Ques. Calculate the average velocity at a particular time of a person when they walk 7 m by 4 seconds and 18 m by 6 seconds in line with the x-axis. (3 Marks)
Ans. It is given that,
- Initial distance traveled xi = 7 m
- Final distance traveled, xf = 18 m
- Initial time interval ti = 4 s
- Final time interval tf = 6 s
Using the Average Velocity Formula,
\(V_{av} = {X_f\,-\,X_i \over t_f\,-\,t_i}\)
Vav = (18−7) / (6−4) = 11/2 = 5.5 m/s.
Thus, the average velocity is 5.5 m/s.
Ques. An object travels 1200 m in 30 s, what is its average velocity? (2 Marks)
Ans. Average Velocity is the rate of change in the displacement of an object in reference to time. Thus,
Velocity = Δt/Δx
Velocity = 1200m/30s = 40m/s
Thus, the velocity of the object is 40m/s.
Ques. The position of the runner as a part-time job is programmed as the x-axis of the linking system. During the 3.00 s period, the runner's position changes from x1 = 50.0 m to x2 = 30.5 m. What was the average velocity of the runner? (3 Marks)
Ans. According to the question,
- Displacement = 30.5 m − 50.0 m = −19.5 m
- Δt = 3.00
Using the Average Velocity Formula,
Vav =Δx/Δt
Vav = −19.5 m/3.00 s
Vav = −6.50 m/s
Thus, the average velocity of the runner is −6.50 m/s.
Ques. The car travels a straight line east of 100 meters in 4 seconds, then west 50 meters in one second. Determine its average velocity. (3 Marks)
Ans. As per the question,
- Distance = 100 meters + 50 meters = 150 meters
- Displacement = 100 meters - 50 meters = 50 meters to the east.
- Time = 4 seconds + 1 second = 5 seconds.
Using the Average Velocity Formula,
\({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\)
Vav = 50 meters/5 seconds = 10 m/s.
Ques. The runner runs around a rectangular track with a length of 50 meters and a width of 20 meters. He circles the rectangle twice, finally running back to where he started. If the total time it takes to run around the track is 100 seconds, determine the average velocity. (3 Marks)
Ans. The perimeter of the Rectangular Track = 2 (50 meters + 20 meters) = 140 meters.
He runs around the rectangle twice, thus, 2 x 140 meters = 280 meters. However, he runs back to the same position at the end, thus,
Displacement= 0 meters. (As the runner returns to the starting position)
Using the Average Velocity Formula,
\({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\)
Vav = 0/100 seconds = 0 m/s.
Ques. An object has a displacement of 304 m north by 180 s. What was the average velocity of the object? (3 Marks)
Ans. Given that
- Displacement = 304 m
- t = 180 s
Using the Average Velocity Formula,
Vav = Displacement / Time
Vav = 304m/180s = 1.7 m/s North.
Ques. Define Average Velocity. (2 Marks)
Ans. Average Velocity is a measure of the total displacement of an object in time ‘t’. It is the ratio of the total displacement of the object to the total time taken. It is denoted by the symbol Vav and is measured in the units of m/s. Average Velocity is a vector quantity as it has both direction as well as magnitude.
Ques. What is the formula for Average Velocity? (3 Marks)
Ans. The Average Velocity Formula is given as
\({Average\,Velocity} = {Total\,Displacement \over Total\,Time}\)
It is also calculated using the formula
\(V_{av} = {X_f\,-\,X_i \over t_f\,-\,t_i}\)
Here, xi and xf are the initial and final velocities and ti and tf are the initial and final time intervals.
Ques. The correct formula for Average Velocity is
(a) v = x/t
(b) v = dx/dt
(c) v = xt/x
(d) v = xt (1 Mark)
Ans. (a) v = x/t
Explanation: Average Velocity is defined as the total change in displacement divided by the total change in time taken by the object.
Check-Out:






Comments